Objectes multimèdia amb l’etiqueta: Facultat de Matemàtiques i Estadística
Resultats de la cerca
Xavier Tort-Martorell (dept. EIO UPC) entrevista a Geoff Vining (dept. Statistics Virginia Tech). Curs Box (2023-2024)
Accés obert
14 de set. 2023
Xavier Tort-Martorell (dept. EIO UPC) entrevista a Geoff Vining (dept. Statistics Virginia Tech). Curs Box (2023-2024)
Acte de presa de possessió de Jordi Guàrdia com a degà de l'FME. Curs Ladyzhenskaya (2022-2023)
Accés obert
28 de març 2023
Tindrà lloc dimarts 28 de març a les 13h30 a la sala d'actes de l'FME, amb l'ordre següent: 13.30 h Obertura de l’acte i salutació a càrrec de Daniel Crespo, rector de la Universitat Politècnica de Catalunya·Barcelona Tech (UPC), qui dona la paraula al degà sortint, Jaume Franch. 13.40 h El rector de la UPC dona la paraula a Ana B. Cortinas, secretària general de la UPC qui fa la lectura del nomenament del degà de la Facultat de Matemàtiques i Estadística (FME). Signatura del llibre de presa de possessió per part de Jordi Guàrdia, Daniel Crespo i Ana B. Cortinas i Lliurament del pin institucional. 13.45 h El rector dona la paraula al degà. Paraules del degà Jordi Guàrdia.Paraules de cloenda a càrrec del rector Daniel Crespo. 14.00 h Gaudeamus Igitur interpretat pel Cor Ol·lari de l’FME 14.05 h Fi de l’acte i trasllat a la sala R (mateixa planta -1) per la copa de cava.
04C-Approximació de funcions amb matemàtiques
Accés obert
2 de nov. 2022
ÉS el TeU DIA!
Actua i fes clic a la ZonaVideo de Càlcul: comprenc, imagino, faig i reviso.
Actua i fes clic a la ZonaVideo de Càlcul: comprenc, imagino, faig i reviso.
Olga A. Ladyzhenskaya: vida y matemáticas de una mujer excepcional. Lliçó inaugural Curs Ladyzhenskaya (2022-2023)
Accés obert
19 d’oct. 2022
Olga A. Ladyzhenskaya: vida y matemáticas de una mujer excepcional. Lliçó inaugural Curs Ladyzhenskaya (2022-2023)
Jornada Ladyzhenskaya FME (Curs 2021-2022)
Accés obert
26 d’abr. 2022
Benvinguda i presentació a càrrec de Jordi Guàrdia, degà de l'FME.
El problema de la velocidad de propagación infinita en las ecuaciones de difusión.
J.M. Mazón (Universidad de Valencia).
Presenta: Albert Mas (Dept. Matemàtiques UPC).
Lliurament premis als guanyadors del Concurs Ladyzhenskaya activitat conjunta de l’assignatura Història de la Matemàtica i la Biblioteca FME.
Characterise and control turbulence in shear flows via nonlinear optimization.
Stefania Cherubini (Polytechnical University of Bari).
Presenta: Joan Sánchez (Dept. Física UPC).
Hopf, Caccioppoli and Schauder, reloaded.
Giuseppe Mingione (University of Parma)
Presenta: Xaiver Cabré (Dept. Matemàtiques UPC).
El problema de la velocidad de propagación infinita en las ecuaciones de difusión.
J.M. Mazón (Universidad de Valencia).
Presenta: Albert Mas (Dept. Matemàtiques UPC).
Lliurament premis als guanyadors del Concurs Ladyzhenskaya activitat conjunta de l’assignatura Història de la Matemàtica i la Biblioteca FME.
Characterise and control turbulence in shear flows via nonlinear optimization.
Stefania Cherubini (Polytechnical University of Bari).
Presenta: Joan Sánchez (Dept. Física UPC).
Hopf, Caccioppoli and Schauder, reloaded.
Giuseppe Mingione (University of Parma)
Presenta: Xaiver Cabré (Dept. Matemàtiques UPC).
Petjades d'una estadística: un tomb per la vida professional. Curs Kolmogórov (2021-2022)
Accés obert
9 de març 2022
En el marc de la celebració del 8M, Dia Internacional de la Dona, l'FME convida Rosa Lamarca, llicenciada en Ciències i Tècniques Estadístiques per la UPC amb més de 20 anys d'experiència en la indústria farmacèutica, que ens farà una presentació del seu bagatge acadèmic i professional amb perspectiva de gènere. Al final s’obrirà un espai de debat i d'intercanvi d'opinions, buscant la participació i la complicitat dels assistents.
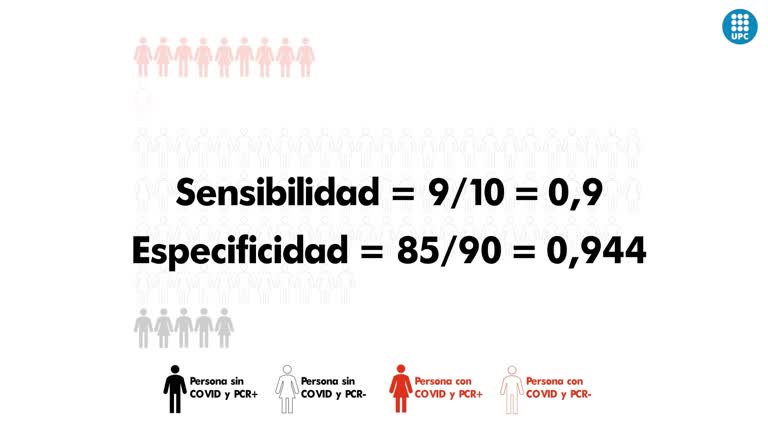
¿Qué conceptos epidemiológicos hay que entender referentes a la COVID-19?
Accés obert
20 de gen. 2022
Explicació divulgativa de conceptes epidemiològics com a prevalença, incidència, sensibilitat, especificitat, entre altres a través d'exemples contextualitzats en la Pandèmia per COVID-19. El coneixement d'aquests conceptes ajuda a tenir una visió més informada de les notícies referents a la propagació d'una epidèmia o relatius a l'eficàcia d'una prova diagnòstica.
Kolmogórov: la K del teorema KAM. Lliçó inaugural Curs Kolmogórov (2021-2022)
Accés obert
25 de nov. 2021
Lliçó inaugural del curs 2021-2022 a càrrec de la professora Tere Martínez-Seara amb el títol "Kolmogórov: la K del teorema KAM". El curs 2021-2022 de l'FME està dedicat a Andrei N. Kolmogórov
Estudia el grau en Ciència i Enginyeria de Dades a la FIB, a l’ETSETB i a l’FME
Accés obert
2 de març 2021
Coneixes el grau en Ciència i Enginyeria de Dades que s’imparteix a la Facultat d’Informàtica de Barcelona, a l’Escola Tècnica Superior d’Enginyeria de Telecomunicació de Barcelona a la Facultat de Matemàtiques i Estadística? Vols saber per què estudiar aquest grau? Descobreix-ho al vídeo!
Estudia el grau en Estadística a l'FME
Accés obert
8 de febr. 2021
Coneixes el grau en Estadística (interuniversitari UB-UPC) que s’imparteix a la Facultat de Matemàtiques i Estadística? Vols saber per què estudiar aquet grau? Descobreix-ho al vídeo!
Estudia el grau en Matemàtiques a l'FME
Accés obert
8 de febr. 2021
Coneixes el grau en Matemàtiques que s’imparteix a la Facultat de Matemàtiques i Estadística? Vols saber per què estudiar aquest grau? Descobreix-ho al vídeo!
